●전기자기학 기초수학:삼각함수,미분 적분.
●삼각함수
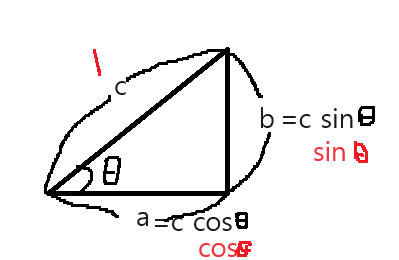
$sin\theta=\frac{b}{c}\to b=c sin\theta$
$cos\theta=\frac{a}{c}\to a=c cos\theta$
$tan\theta=\frac{b}{a}=\frac{sin\theta}{cos\theta}$
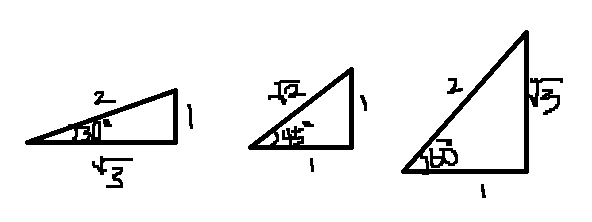
| $\theta$ | $0^{\circ}$ | $30^{\circ}$ | $45^{\circ}$ | $60^{\circ}$ | $90^{\circ}$ |
| $sin\theta$ | 0 | $\frac{1}{2}$ | $\frac{1}{\sqrt2}$ | $\frac{\sqrt3}{2}$ | 1 |
| $cos\theta$ | 1 | $\frac{\sqrt3}{2}$ | $\frac{1}{\sqrt2}$ | $\frac{1}{2}$ | 0 |
| $tan\theta$ | 0 | $\frac{1}{\sqrt3}$ | 1 | $\sqrt3$ | $\infty$ |
●피타고라스 정리
$a^2+b^2=c^2$
※위 삼각함수 그림에서 c=1이라면 $b=sin\theta/,/,c=cos\theta$가 된다. a와b를 대입하면
$c^2cos^2\theta+c^2sin^2\theta=c^2$
${\color{Red}cos^2\theta+sin^2\theta=1}$
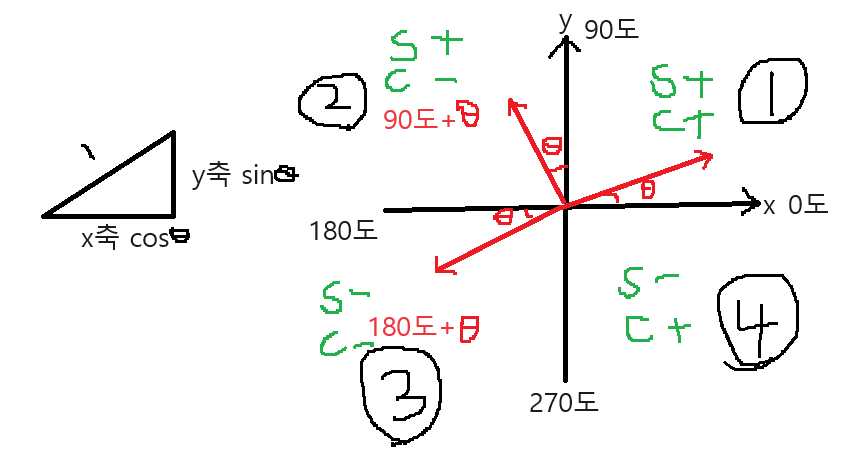
*위의 삼각형에서 sin은 y축이고, cos은 x축이다. 그러므로 각 사분면의 sin과 cos의 부호는 위의 그림과 같다.
*먼저 부호부터 정리를 해야한다.
| 1사분면 | $sin\theta$(+) |
| $cos\theta$(+) | |
| 2사분면 | $sin\theta$(+) |
| $cos\theta$(-) | |
| 3사분면 | $sin\theta$(-) |
| $cos\theta$(-) | |
| 4사분면 | $sin\theta$(-) |
| $cos\theta$(+) |
*2사분면일 때는 부호는 그대로, sin과 cos이 바뀐다.
${\color{Red}sin(90^{\circ}+\theta)=+cos\theta}$
${\color{Red}cos(90^{\circ}+\theta)=-sin\theta}$
*3사분면일 때는 모두 다 그대로 간다.
$sin(180^{\circ}+\theta)=-sin\theta$
$cos(180^{\circ}+\theta)=-cos\theta$
보기)
$sin120^{\circ}\to sin(90^{\circ}+30^{\circ})\to +cos30^{\circ}=\frac{\sqrt3}{2}$
$cos240^{\circ}\to cos(180^{\circ}+60^{\circ})\to-cos60^{\circ}=-\frac{1}{2}$
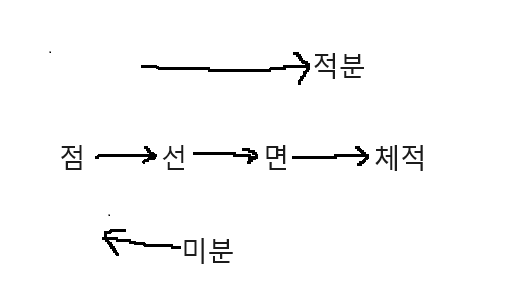
●미분과 적분
*미분의 미는 미세하다는 의미이고, 분은 나누다라는 뜻이다.
*미분:미세하게 나눈 부분→기울기,변하량
*적분의 적은 쌓는다는 의미이다.
*적분:미세한 부분을 쌓는 것 →면적, 체적
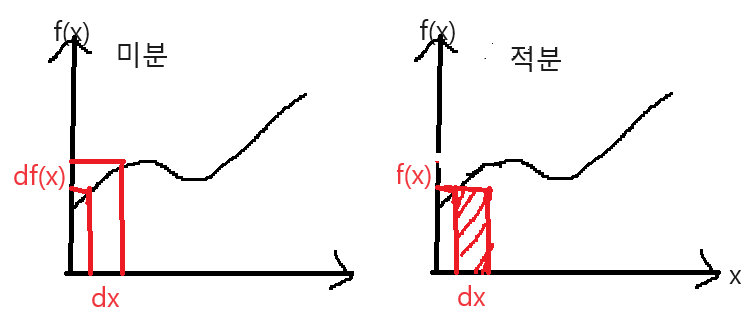
● 미분:$\frac{df(x)}{dx}\to\frac{d}{dx}f(x)\to f(x)'$
*$\frac{d}{dx}x^n\,\,\to n.x^{n-1}$
$x^{0}$의 미분=1이고, 상수의 미분=0
예)$x^3\to 3x^2 \to 3.2x^1 \to 6\to 0$(연속해서 미분한 것)
● 적분:$\int f(x).dx$
예)$\int6x^0.dx\to 6.\frac{1}{0+1}x^1\to 6.\frac{1}{2}x^2\to3.\frac{1}{3}x^3$(연속해서 적분한 것)
● 로그함수
*$\frac{1}{x}=x^{-1}$
※$2^x=3\to x=log_{2}^{3}$
※$10^x=3\to x=log_{10}^{3}$를 상용로그라 하고 $=log3$이라 한다.
※$e^x=3\to x=log_{e}^(3)=l_n3$e는 자연상수.
$\int\frac{1}{x}dx=l_nx=log_{e}^{x}$
$l_n1=0$
$l_nab=l_na+l_nb$
$l_n\frac{a}{b}=l_na-l_nb$
$l_nb^{a}=a.l_nb$
● 삼각함수 미분
$\frac{d}{dx}sin x\to cos x$
$\frac{d}{dx}cos x\to (-)sin x$
● 삼각함수 적분
$\int sin x.dx\to(-)cos x$
$\int cos x.dx\to sin x$
'전기(산업)기사필기 > 전기자기학' 카테고리의 다른 글
| 전기력선,전기력서의 특징,전기력선의 방정식,가우스법칙,포아송방정식,전속,전속밀도,전기자기학(전기기사) (2) | 2023.12.20 |
|---|---|
| 정전계,쿨롱의 법칙,정전력,전계의 세기,전위,등전위면,도체의 성질,전기자기학(전기기사) (0) | 2023.12.10 |
| 원자의 구조,전하량,전기자기학(전기기사) (0) | 2023.12.01 |
| 벡터의 연산,더하기,빼기,내적,외적,기울기,발산,회전,전기자기학(전기기사) (0) | 2023.11.10 |
| 벡터와스칼라,성분벡터(기본벡터),단위벡터,직교좌표,직각좌표,원통좌표,구형좌표,전기자기학(전기기사) (0) | 2023.11.07 |



